8: Appendix Joint Costs
- Page ID
- 51377
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Appendix: Making Decisions Involving Joint Costs
Learning Objectives
- Analyze the impact that joint costs have on decision making.
Question: When two or more products are produced from a single input, these products are called joint products. For example, lumber companies often must deal with joint products (different types of lumber) resulting from one input (a log). How do the concepts of joint products and joint costs help a lumber company establish a cost for each of its products?
Answer: Suppose Oregon Lumber Company takes a log (the single input) and mills it into two types of products: high quality Grade A lumber, and lower quality Grade B lumber. Grade A lumber and Grade B lumber are examples of joint products, and the cost of the logs and related manufacturing process costs are examples of joint costs.
The following represents the information for Oregon Lumber for the month of June. Joint costs for the month total $250,000. Notice that the split-off point is the point at which identifiable products emerge from the production process. The issue is how to allocate joint costs—the $250,000 in production costs incurred prior to the split-off point—to the resulting joint products.
Two methods are commonly used to allocate these joint costs to the joint products: the physical quantities method and the sales value method. We discuss each of these methods next.
The Physical Quantities Method
Question: The physical quantities method allocates joint costs based on a physical measure of output. Assume Oregon Lumber produces 600,000 board feet of Grade A lumber and 200,000 board feet of Grade B lumber during June. How would Oregon Lumber use this information to allocate $250,000 in joint production costs to each grade of lumber?
Answer: Oregon Lumber would allocate 75 percent of the joint costs to Grade A lumber (75 percent = 600,000 Grade A board feet ÷ 800,000 total board feet), and 25 percent of the joint costs to Grade B lumber.
Grade A allocation:
$187,500 allocation = $250,000 joint costs × (600,000 Grade A board feet ÷ 800,000 total board feet)
Grade B allocation:
$62,500 allocation = $250,000 joint costs × (200,000 Grade B board feet ÷ 800,000 total board feet)
Figure A “Joint Product Profitability for Oregon Lumber Company: Physical Quantities Method” presents the profitability of each joint product for the month using the physical quantities method assuming Grade A lumber sells for $0.40 per board foot and Grade B lumber sells for $0.30 per board foot.
Figure A Joint Product Profitability for Oregon Lumber Company: Physical Quantities Method
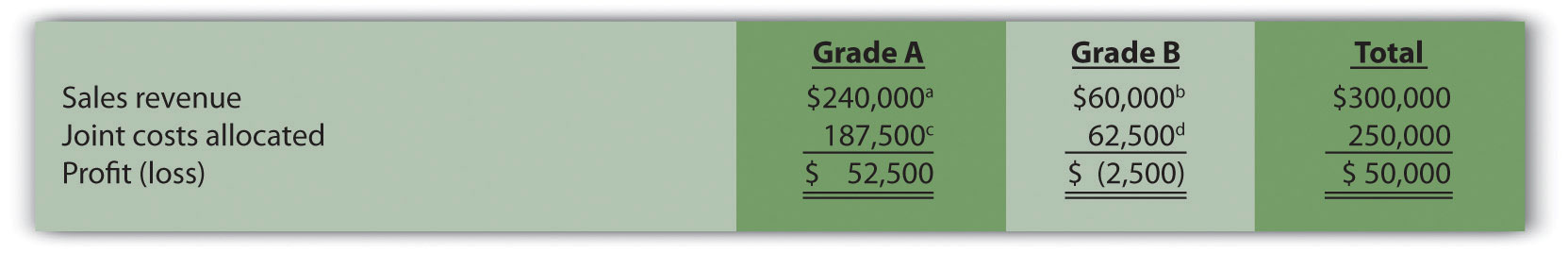
a $240,000 = $0.40 per board foot × 600,000 Grade A board feet.
b $60,000 = $0.30 per board foot × 200,000 Grade B board feet.
c $187,500 = $250,000 joint costs × (600,000 Grade A board feet ÷ 800,000 total board feet).
d $62,500 = $250,000 joint costs × (200,000 Grade B board feet ÷ 800,000 total board feet).
Although Grade B lumber appears to be unprofitable, elimination of Grade B lumber sales would not increase overall profit for Oregon Lumber. Grade B lumber contributes $60,000 to covering joint costs. Thus elimination of Grade B lumber sales would result in a decrease in overall profit of $60,000. The $62,500 in joint cost allocated to Grade B lumber would simply be reallocated to Grade A lumber.
The Sales Value Method
Question: A different approach to allocating joint costs to joint products is the sales value method , which allocates joint costs based on the relative sales value of each product at the split-off point. How would Oregon Lumber allocate joint production costs using this method?
Answer: Because sales revenue totals $240,000 for Grade A lumber and $60,000 for Grade B lumber, 80 percent of the joint costs are allocated to Grade A lumber (80 percent = $240,000 Grade A revenue ÷ $300,000 total revenue), and 20 percent of the joint costs are allocated to Grade B lumber:
Grade A allocation:
$200,000 allocation = $250,000 joint costs × ($240,000 Grade A sales value ÷ $300,000 total sales value)
Grade B allocation:
$50,000 allocation = $250,000 joint costs × ($60,000 Grade B sales value ÷ $300,000 total sales value)
Figure B “Joint Product Profitability for Oregon Lumber Company: Sales” presents the profitability of each joint product for the month using the sales value method, again assuming Grade A lumber sells for $0.40 per board foot, and Grade B lumber sells for $0.30 per board foot.
Figure B Joint Product Profitability for Oregon Lumber Company: Sales
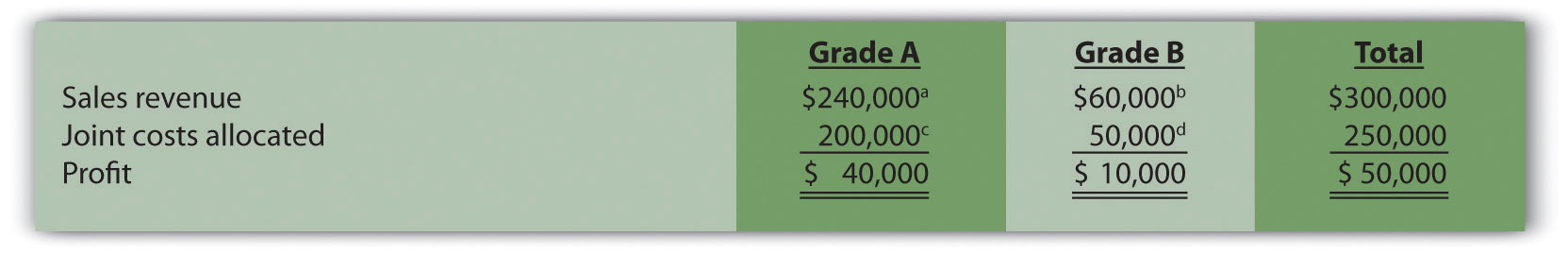
a $240,000 = $0.40 per board foot × 600,000 Grade A board feet.
b $60,000 = $0.30 per board foot × 200,000 Grade B board feet.
c $200,000 = $250,000 joint costs × ($240,000 Grade A sales value ÷ $300,000 total sales value).
d $50,000 = $250,000 joint costs × ($60,000 Grade B sales value ÷ $300,000 total sales value).
The sales value method assumes that profit as a percent of sales will remain the same across all products. For example, Figure B shows that Grade A lumber has a profit margin ratio of 16.67 percent (= $40,000 profit ÷ $240,000 sales), as does Grade B lumber (= $10,000 profit ÷ $60,000 sales). This method also ensures that joint costs allocated to each product will not exceed sales revenue for each product (unless total joint costs are higher than total revenue).
As you review both methods and the results, notice that the total column for both methods of joint cost allocation is the same. The issue is not with the overall results. The issue is how to allocate joint costs to each joint product.
Deciding Whether to Process Further
Question: Assume Oregon Lumber Company has the option of processing Grade B lumber further into a finished product by sanding the lumber and painting it with primer. The sanded and painted Grade B lumber sells for $0.45 per board foot rather than $0.30 for the unfinished Grade B lumber. The additional cost to sand and paint the Grade B lumber is $0.05 per board foot. Should Oregon Lumber process Grade B lumber further into finished lumber?
Answer: The answer depends on whether the additional revenue exceeds the additional cost of processing Grade B lumber further. Since the additional revenue of $0.15 per board foot (= $0.45 finished price − $0.30 unfinished price) is greater than the additional $0.05 per board foot processing cost, Oregon Lumber should process the Grade B lumber further into finished lumber. Profit increases $0.10 per board foot as a result of processing further (= $0.15 additional revenue − $0.05 additional cost).
Oregon Lumber will decide whether or not to process Grade B lumber further regardless of how joint costs are allocated to Grade A and Grade B lumber. In a sense, joint costs are sunk costs with respect to this decision, and will not influence future processing decisions. Thus joint costs incurred prior to the split-off point are irrelevant to the decision whether to process further after the split-off point.
Key Takeaways
- Two or more products made from a single input are called joint products. The costs of the single input and related manufacturing process costs must be allocated to each of the joint products. The physical quantities method allocates joint costs based on a physical measure of output (e.g., pounds or yards of material). The sales value method allocates joint costs based on the relative sales value for each of the joint products. Regardless of the allocation method used, total joint costs and total profit remain the same. Companies must often decide whether to process a joint product further. If as a result of processing the product further, additional sales revenue exceeds additional costs, the wise decision is to process further.
Check Yourself
Fresh Veggies, Inc., purchased 10,000 pounds of fresh apples from a local grower for $4,000. The apples were separated into high-quality Grade A apples (3,000 pounds) and lower-quality Grade B apples (7,000 pounds). Fresh Veggies sells Grade A apples for $0.80 per pound and Grade B apples for $0.50 per pound.
- Allocate joint costs to each product using the physical quantities method (pounds), and calculate the profit or loss for each product.
- Allocate joint costs to each product using the relative sales value method, and calculate the profit or loss for each product.
- Assume Grade B apples can be processed further into dried apple slices for an additional $0.20 per pound. Customers are willing to pay $0.65 per pound for dried apple slices. Should Fresh Veggies, Inc., process the Grade B apples further?
Solution
-

a $2,400 = $0.80 per pound × 3,000 pounds of Grade A apples.
b $3,500 = $0.50 per pound × 7,000 pounds of Grade B apples.
c $1,200 = $4,000 joint costs × (3,000 pounds of Grade A apples ÷ 10,000 total pounds).
d $2,800 = $4,000 joint costs × (7,000 pounds of Grade B apples ÷ 10,000 total pounds).
-
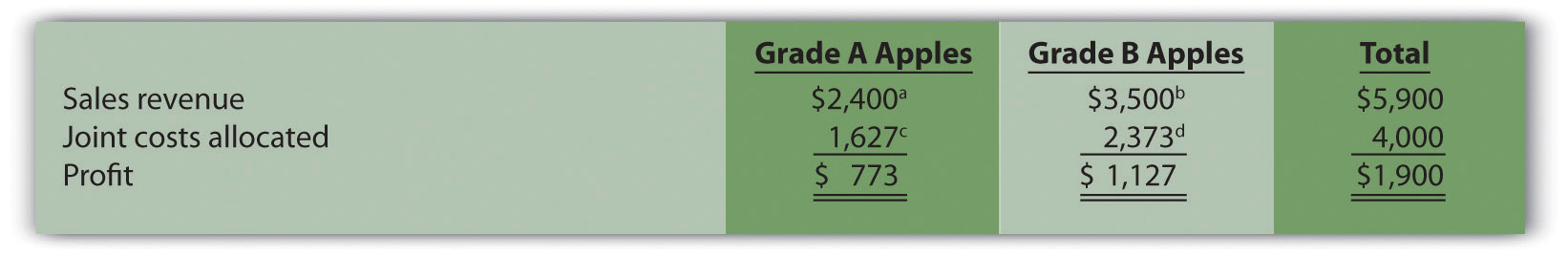
a $2,400 = $0.80 per pound × 3,000 pounds of Grade A apples.
b $3,500 = $0.50 per pound × 7,000 pounds of Grade B apples.
c $1,627 (rounded) = $4,000 joint costs × ($2,400 Grade A sales value ÷ $5,900 total sales value).
d $2,373 (rounded) = $4,000 joint costs × ($3,500 Grade B sales value ÷ $5,900 total sales value).
- Because the additional revenue of $0.15 per pound (= $0.65 price with further processing − $0.50 without further processing) is less than the additional $0.20 per pound processing cost, Fresh Veggies should not process the Grade B apples further into dried apples. Profit decreases $0.05 per pound (= $0.20 additional cost − $0.15 additional revenue) as a result of processing further.

